- Before finally concluding on the period of 311 +/- 1 days, we have to do one last check : we have to demonstrate that this period can not be the result of aliasing, i.e. a false peak caused by the observing rate.
We will create a Spectral Window which exactly calculates the pattern caused by the structure of gaps in the observations. It is not a true Fourier spectrum for R Leo, but it indicates what peaks in a Period window are artifacts of the 'sampling rate'. Since R Leo observations become impossible each year around the same time, it is very likely that aliasing with a period of 365 days will be present.
- Select Spectral Window in the Period analysis menu of the Observations Window, to display the Spectral Window dialog box. It is very similar to the Period Determination box used in previous steps of this tutorial. Leave all entries as is and press Apply to calculate the Spectral Window.

- This creates a new Period window with caption "PerWin - SpectralWindow #1 for ObsWin #1". We easily recognise a peak near 365 days, as predicted. We observe no peak near 311 days, so the R Leo period found in this tutorial is not the result of any 'observations sampling rate'.
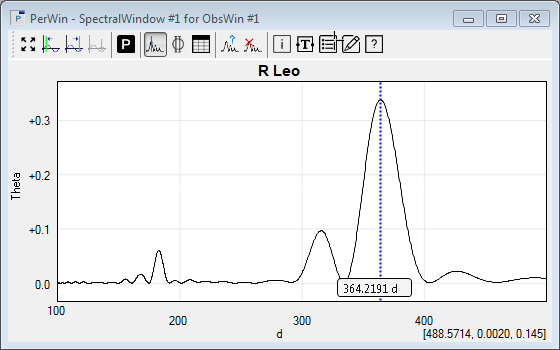
We can now safely conclude - on the basis of the AAVSO sample of observations we used - that R Leo has a period of 311 +/- 1 days.
Advanced exercise
Make yourself familiar with the Lightcurve Workbench of the R Leo Observations window. Select the Binning tab and create a new binned ObsWin by binning all R Leo observations in bins with a size of "10 days". The new ObsWin will have approximately 310 observations. Rerun your Lomb-Scargle and ANOVA period analyses on the new ObsWin. You will obtain values of resp. 310.9 +/- 3.7 days and 310.9 +/- 0.6 days. The much smaller data set produces values in good agreement with the large data set of nearly 15,000 observations we have been using so far. Finally, produce a Spectral Window using a Range going from 5 to 500 days. Your Spectral Window will now clearly show a dominant peak at 10 days, caused by the sampling (binning) value you have used to produce the R Leo binned ObsWin. The alias near 365 days is also still present, but is much less pronounced than the 10 days alias.
|